Difference between revisions of "Topology"
From Design Computation
(→Cross-Link) |
|||
| (4 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
[[Category:Key Page]] | [[Category:Key Page]] | ||
| − | In mathematics, topology (from the Greek τόπος, place, and λόγος, study) is concerned with the properties of space that are preserved under continuous deformations, such as stretching, crumpling and bending, but not tearing or gluing. This can be studied by considering a collection of subsets, called open sets, that satisfy certain properties, turning the given set into what is known as a topological space. Important topological properties include connectedness and compactness. | + | [[Category:Cover Term]] |
| + | |||
| + | [[Image:Möbius strip.jpg|thumb|240px|[[Möbius strip]]s, which have only one surface and one edge, are a kind of object studied in topology.]] | ||
| + | |||
| + | In [[mathematics]], topology (from the Greek τόπος, place, and λόγος, study) is concerned with the properties of space that are preserved under continuous deformations, such as stretching, crumpling and bending, but not tearing or gluing. This can be studied by considering a collection of subsets, called open sets, that satisfy certain properties, turning the given set into what is known as a topological space. Important topological properties include connectedness and compactness. | ||
=Cross-Link= | =Cross-Link= | ||
| + | *[[Mathematics]] | ||
| + | *[[Craftmanship]] | ||
| + | |||
| + | =References= | ||
Latest revision as of 17:12, 9 January 2018
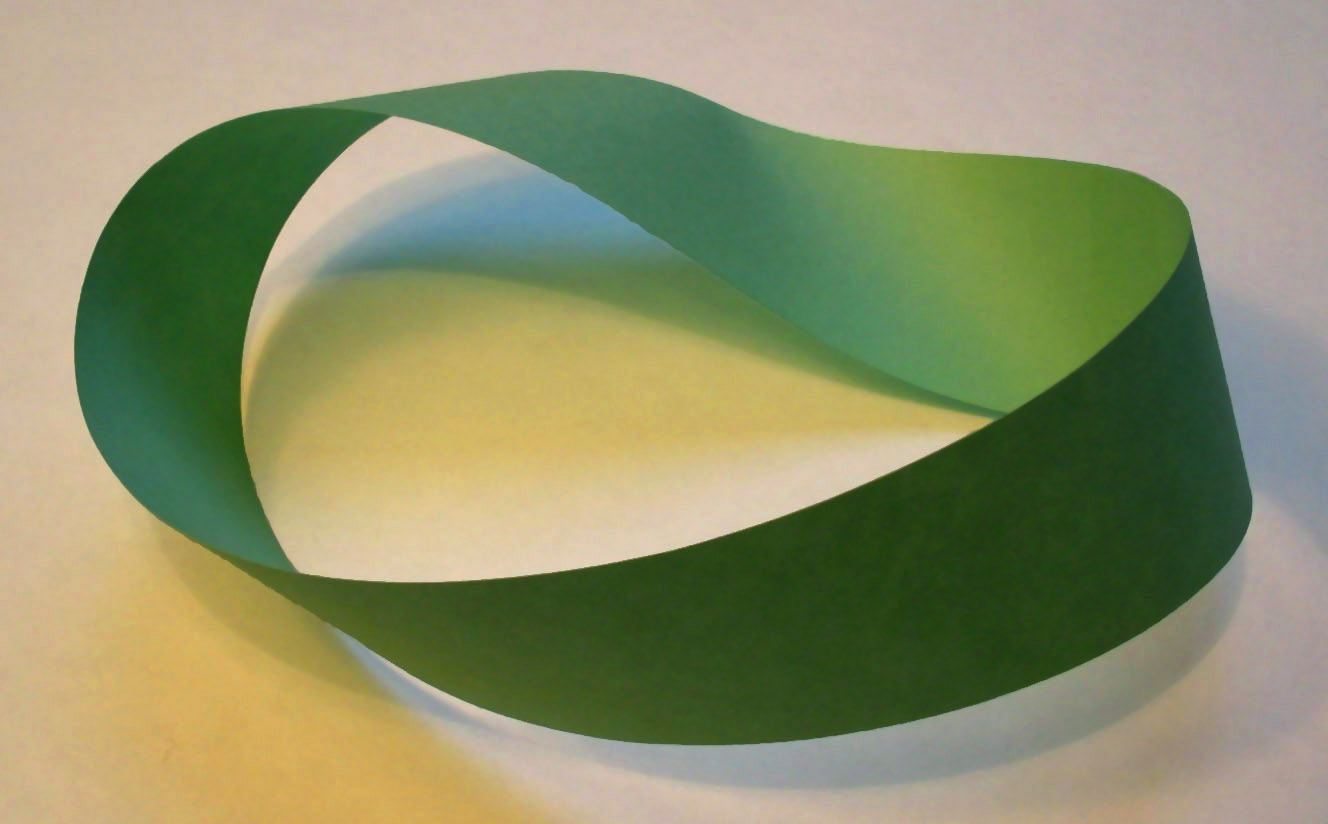
Möbius strips, which have only one surface and one edge, are a kind of object studied in topology.
In mathematics, topology (from the Greek τόπος, place, and λόγος, study) is concerned with the properties of space that are preserved under continuous deformations, such as stretching, crumpling and bending, but not tearing or gluing. This can be studied by considering a collection of subsets, called open sets, that satisfy certain properties, turning the given set into what is known as a topological space. Important topological properties include connectedness and compactness.